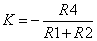OKAWA Electric Design
English  |  Japanese

3rd order Multiple feedback Low-pass Filter Design Tool

This page is a web calculator that design a 3rd order Multiple feedback low-pass filter. Use this utility to simulate the Transfer Function for filters at a given frequency, damping ratio ζ or values of R and C. The response of the filter is displayed on graphs, showing Bode diagram, Nyquist diagram, Impulse response and Step response.

Sample calculation

Calculate the transfer function for 3rd order Multiple feedback low-pass filter with R and C values

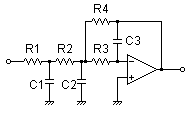
Vi→ →Vo
Transfer function:
    Transfer function of 3rd order Multiple feedback low-pass filter


2nd order Multiple feedback low-pass filter tool, use also.
R1=Ω C1=F
R2=Ω C2=F
R3=Ω C3=F
R4=Ω
p:pico, n:nano, u:micro, k:kilo, M:mega

Frequency analysis

Bode diagram
    Phase  Group delay
Nyquist diagram
Pole, zero
Phase margin
Oscillation analysis
Analysis on frequency range:
  f1=∼f2=[Hz] (optional)

Transient analysis

Step response
Impulse response
Overshoot
Final value of the step response
Analysis on time range:
  0∼[sec] (optional)


Calculate the R and C values for the Multiple feedback filter at a given frequency and damping ratio ζ

Vi→ →Vo
※ 3rd order filters are usually made from combinations of 1st and 2nd order filters following the equivalent block diagram.


Equivalent block diagram:

Vi→2πfc1
 s+2πfc1 		→K(2πfc2)2
 s2+2ζ(2πfc2)s+(2πfc2)2 		→Vo


Transfer function block diagram:




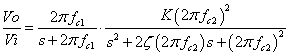
Filter gain at f=0Hz:


Filter gain at f=0Hz:
  K=[Times] (K<0)

Select filter type

Set parameters of the equivalent block diagram
1st order filter:
  fc1=Hz
2nd order filter:
  fc2=Hz Damping ratio ζ=

Butterworth filter
  Cut-off frequency fc=Hz
Chebyshev filter
  Characteristic frequency
  fc=Hz
  Gain ripple
  gr=dB
Bessel filter
  Characteristic frequency
  fc=Hz

C1 = F C2 = F
C1, C2 is optional. But when setting these capacitances, C1 and C2 of both are needed.

Select Capacitor Sequence:
Select Resistor Sequence:

Frequency analysis

Bode diagram
    Phase  Group delay
Nyquist diagram
Pole, zero
Phase margin
Oscillation analysis
Analysis on frequency range:
  f1=∼f2=[Hz] (optional)

Transient analysis

Step response
Impulse response
Overshoot
Final value of the step response
Analysis on time range:
  0∼[sec] (optional)


Filter tools

  1. RC LPF
  2. RC HPF
  3. LR LPF
  4. LR HPF
  5. RLC LPF
  6. RLC HPF
  7. RLC BPF
  8. RLC BEF
  9. Sallen-Key LPF
  10. Sallen-Key HPF
  11. 3rd order SallenKeyLPF
  12. 3rd order SallenKeyHPF
  13. Multiple feedback LPF
  14. Multiple feedback HPF
  15. Multiple feedback BPF
  16. 3rd order Multiple feedback LPF
  17. 3rd order Multiple feedback HPF
  18. TwinT notch
  19. CR 2nd order LPF,HPF,BPF
  20. Active filter

Filter index